Finding the Equation of a Straight Line

To find the equation of a straight line, we use the formula:

$$ y = mx + c $$

🌟 The Formula to Find the Slope m:

$$ m = \frac{y_2\, -\, y_1}{x_2 \,-\, x_1} $$

• We are given two points on the line:

$$
\begin{aligned}
P_1 &= (2, 3) \\
P_2 &= (4, 7)
\end{aligned}
$$

• Substitute the values into the formula:

$$ m = \frac{7\, – \,3}{4\, – \,2} = \frac{4}{2} = 2 $$

• So, the slope is $m = 2$.

Now that we’ve found the slope $m = 2$, we plug it into the equation $y = mx + c$.

This gives us:

$$y=2x+c$$

🌟 Finding c Using a Point on the Line:

• • Let’s use the point $P_2 = (4, 7)$. Substitute $x = 4$ and $y = 7$ into the equation:

$$
7 = 2 \times 4 + c
$$

• • So the y-intercept is $c = -1$.

🌟 Determining the Equation of the Straight Line:

• • Now substitute $m = 2$ and $c = -1$ back into:

$$y = mx + c$$

• • So, the equation of the line passing through the points $(2, 3)$ and $(4, 7)$ is:

$$
y = 2x\, – \,1
$$

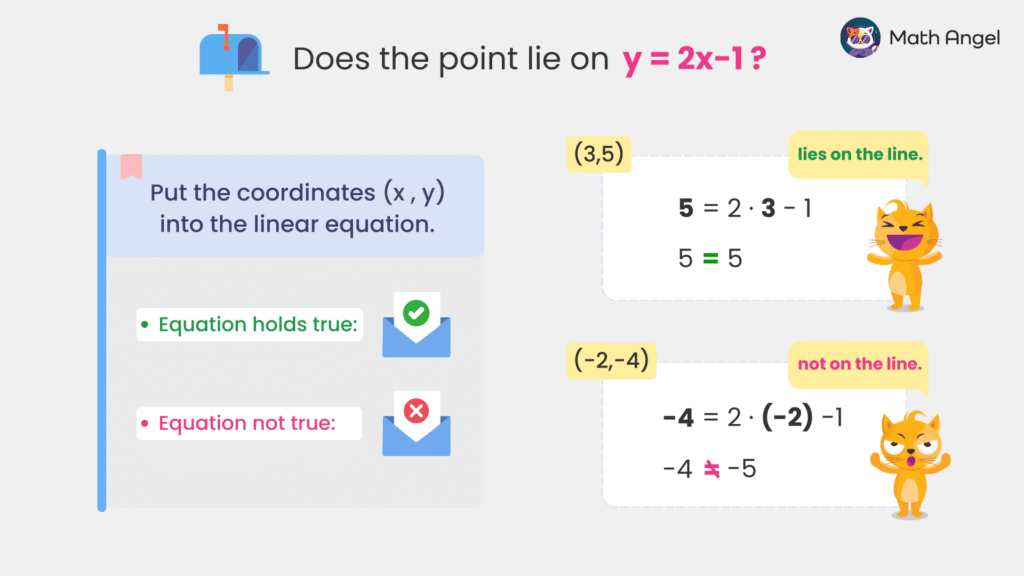
To check if a point lies on a line, substitute the x-value or the y-value of the point into the equation. If the other value you get matches the point’s coordinates, then the point lies on the line.

🏆 Example 1: Check if the point $(3, 5)$ lies on the line $y = 2x\, – \,1$.

• Substitute $x = 3$ into the equation:
  $$y = 2 \times 3\, – \,1 = 5$$
• Since $y = 5$, which matches the $y$-value of the point, the point $(3, 5)$ lies on the line $y = 2x\, – \,1$.

🏆 Example 2: Check if the point $(-2, -4)$ lies on the line $y = 2x\, – \,1$.

• Substitute $x = -2$ into the equation:
  $$y = 2 \times (-2)\, – \,1 = -5$$
• But the point’s $y$-value is $-4$, not $-5$. So, the point $(-2, -4)$ is not on the line $y = 2x\, – \,1$.