Pythagoras Theorem

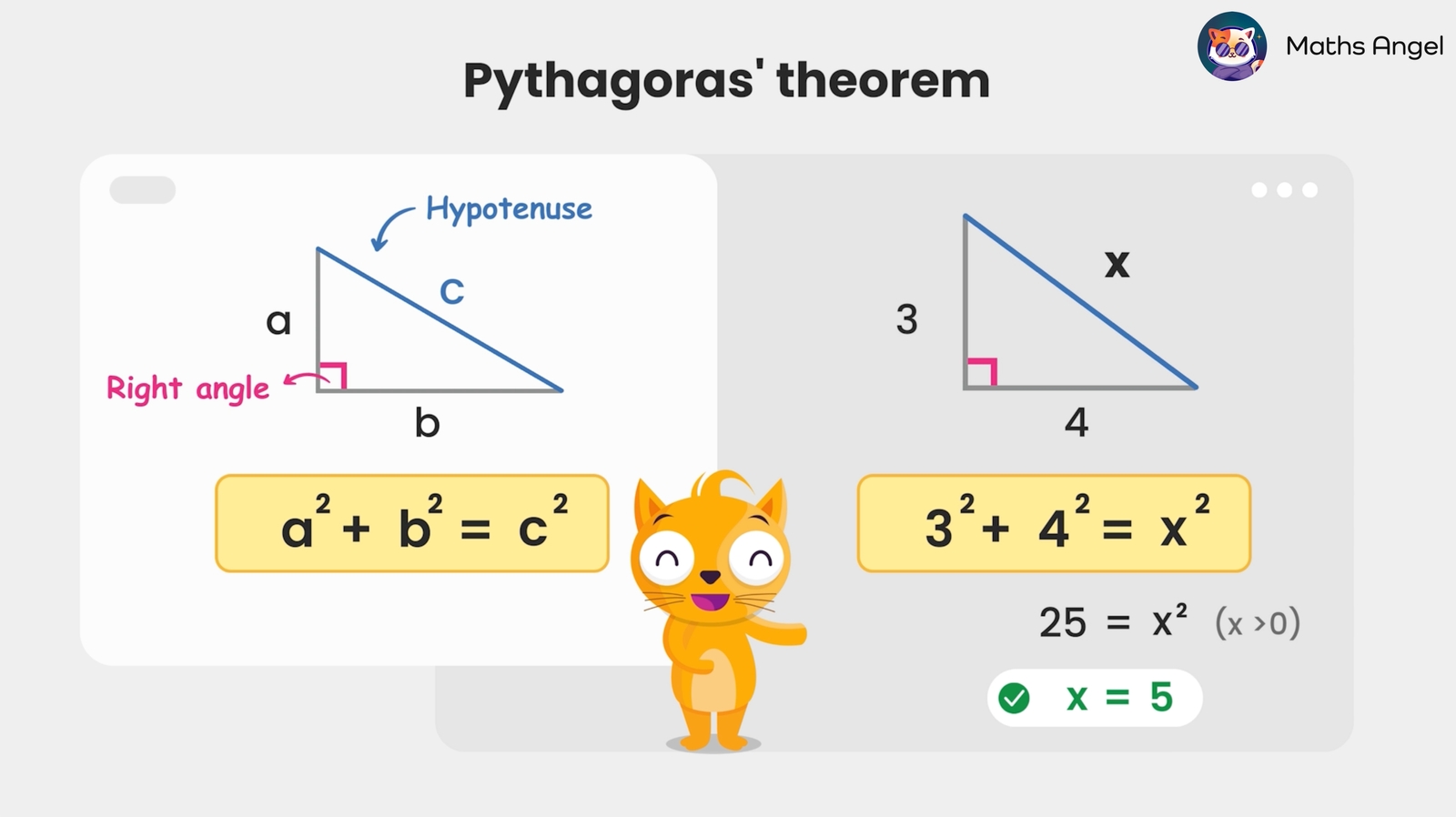
Pythagoras’ Theorem states that in a right-angled triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.

The formula is:

$$ a^2 + b^2 = c^2 $$

where:

• $a$ and $b$ are the two shorter sides (legs).
• $c$ is the hypotenuse (the longest side opposite the right angle).

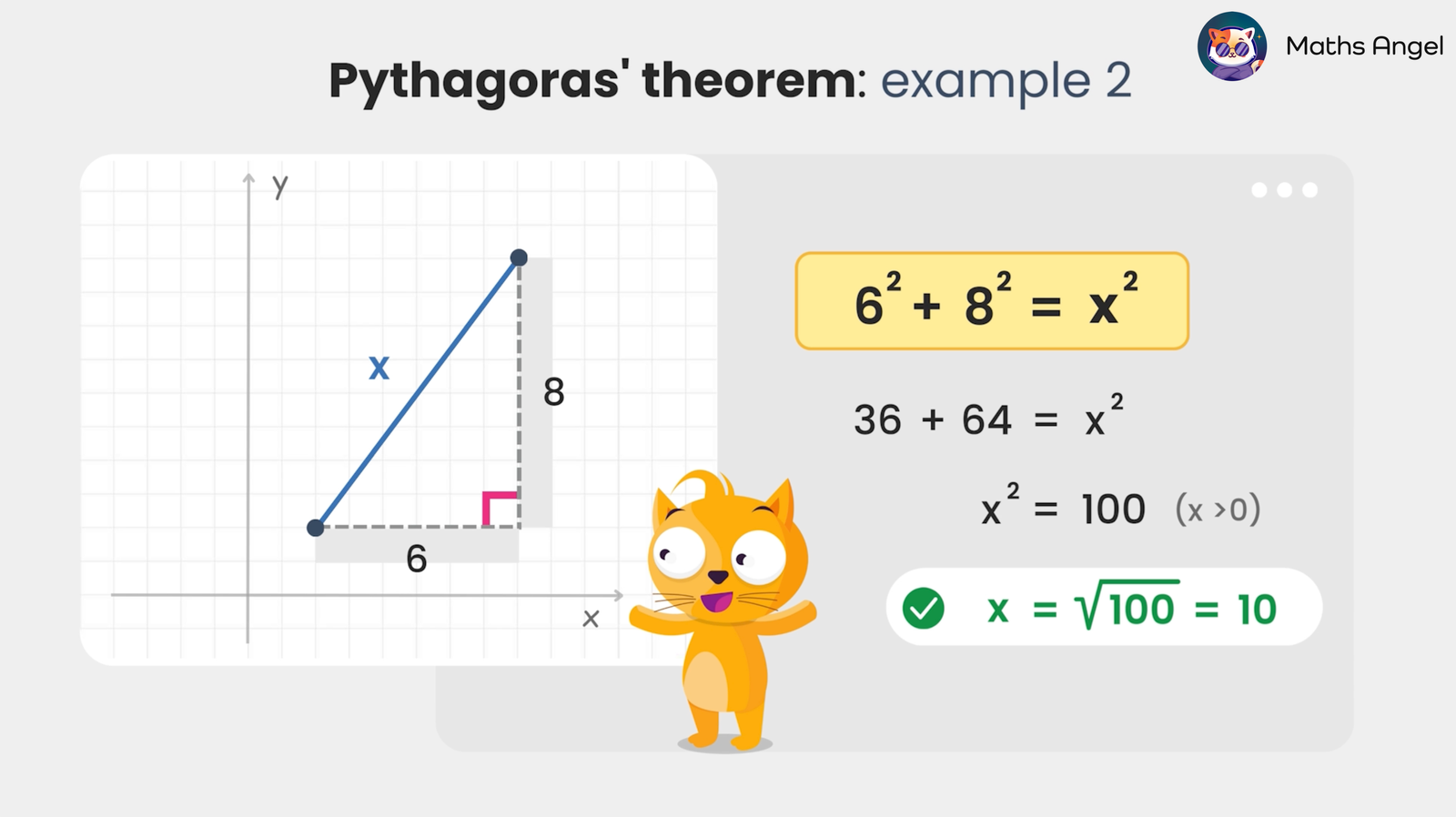
You can use Pythagoras’ Theorem to find the missing side in a right-angled triangle. 

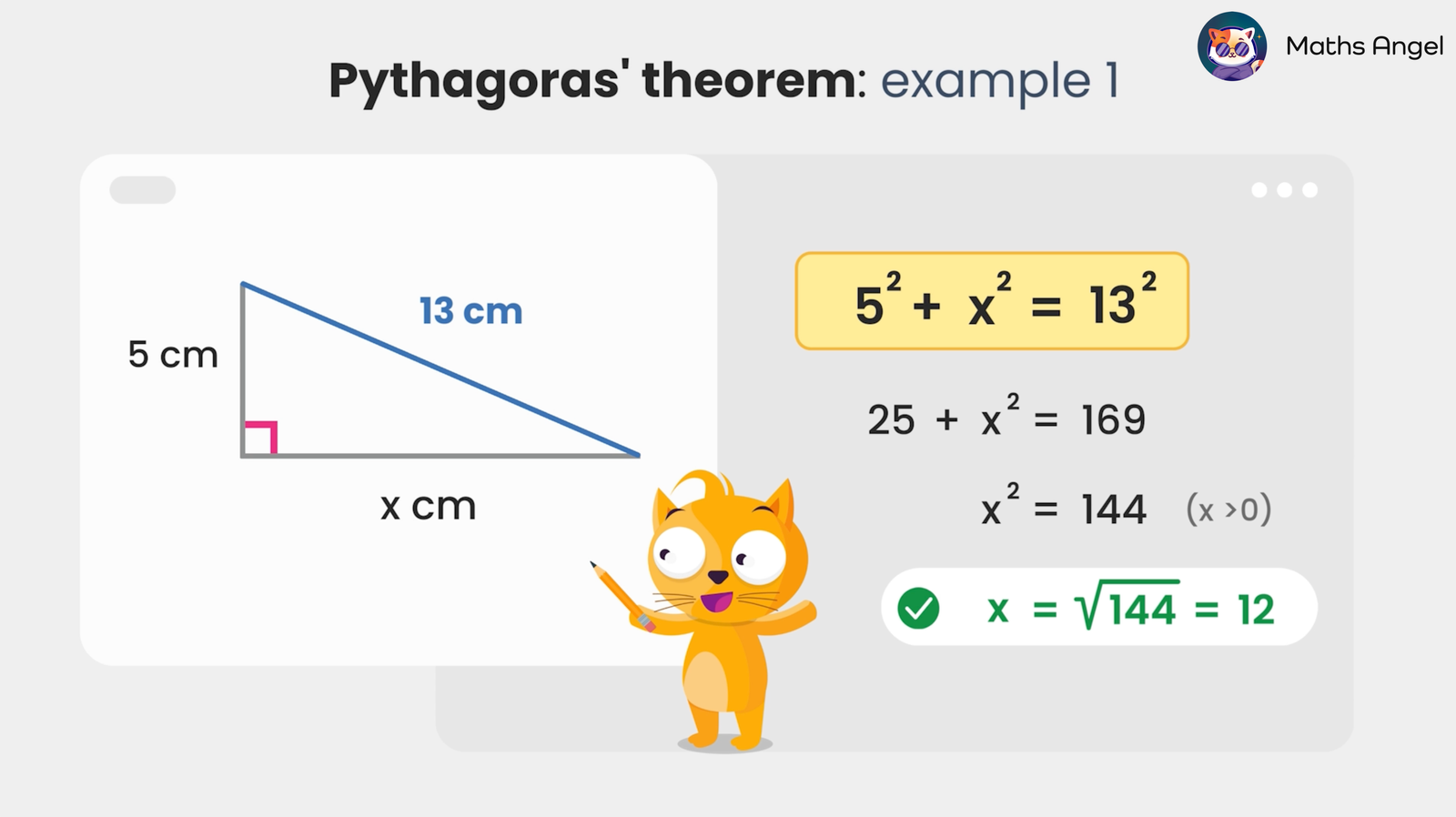
For example, given a right-angled triangle with one side of 5 and hypotenuse of 13. Find the other side $x$.

• Step 1: Use Pythagoras’ Theorem: $$ 5^2 + x^2 = 13^2 $$
• Step 2: Simplifying the equations: 

$$
\begin{aligned}
25 + x^2 &= 169 \\
x^2 &= 144
\end{aligned}
$$

• Step 3: Take the positive square root (since hypotenuse $x$ cannot be negative):

$$ x = \sqrt{144} = 12 $$

Thus, the other side is 12.

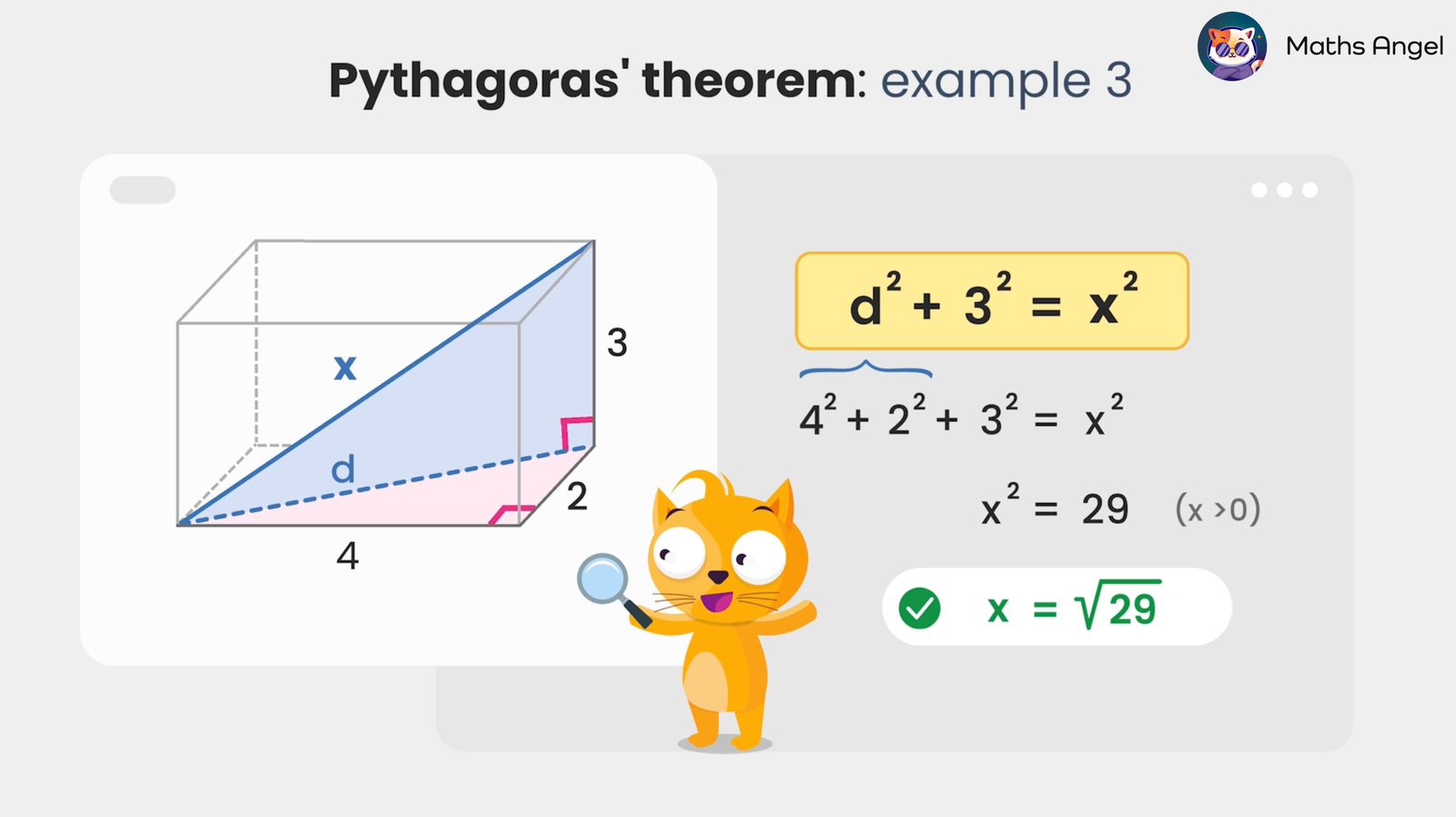
Pythagoras’ Theorem can also be used in three dimensions to find the space diagonal of a cuboid—the longest diagonal. The trick is to apply Pythagoras’ Theorem twice.

For example, given a cuboid with side lengths 4, 2, and 3, find the space diagonal $x$:

• Step 1: First, find the diagonal of the base $d$ using Pythagoras’ Theorem:

\begin{aligned}
d^2 &= 4^2 + 2^2 \\[0.5em]
d^2 &= 16 + 4 \\[0.5em]
d^2 &= 20
\end{aligned}

• Step 2: Now, use Pythagoras’ Theorem again to find the full space diagonal $x$:

$$ d^2 + 3^2 = x^2 $$

$$ 20 + 9 = x^2 $$

$$ x = \sqrt{29} $$

Thus, the space diagonal of the cuboid is $\sqrt{29}$.