Gradient and Y-Intercept in Linear Equations
🎬 Video: Understanding y = mx + c
What are Linear Equations? (0:01)
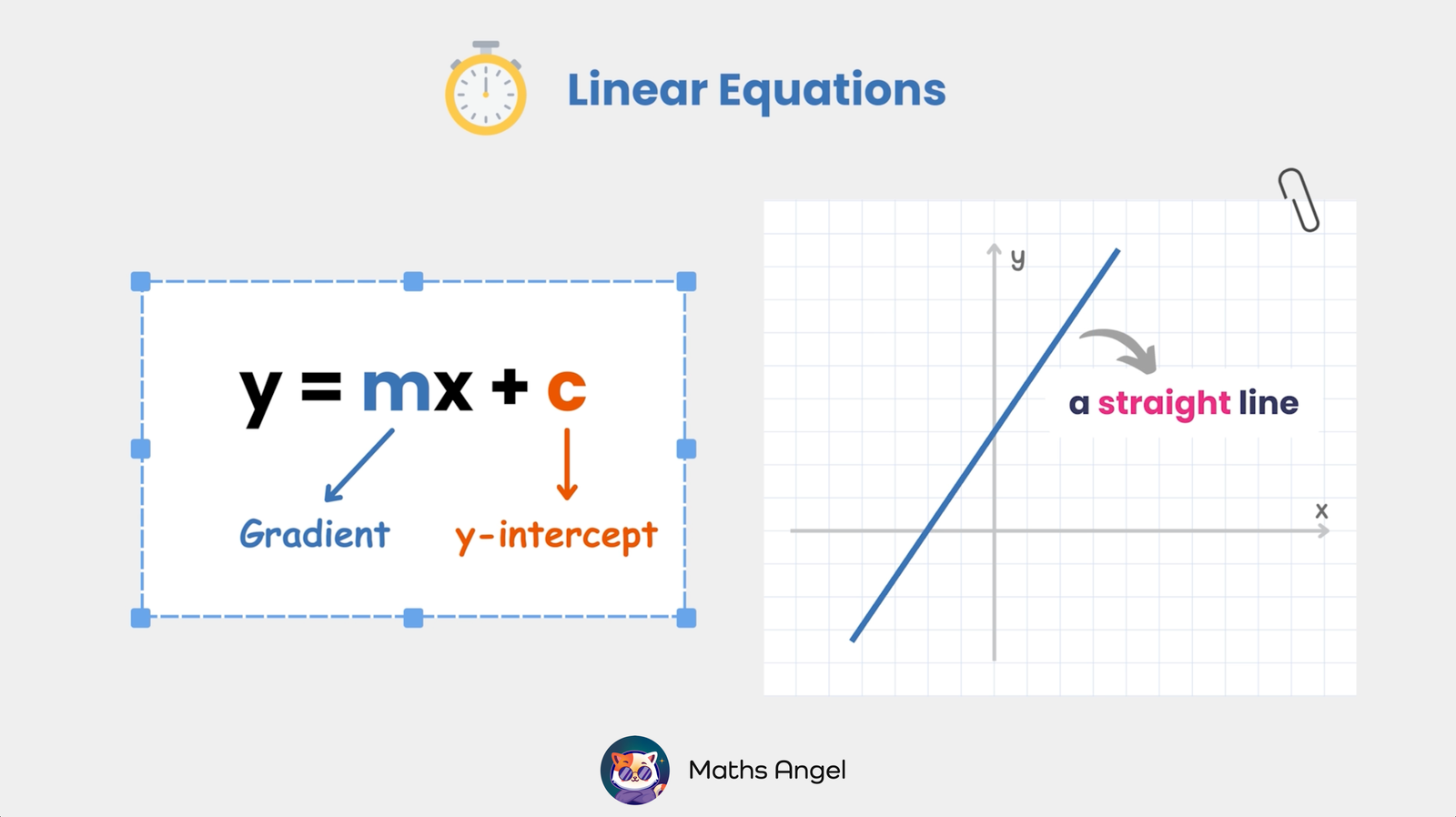
A linear equation is an equation that represents a straight-line relationship between variables. It follows the form:
$$ y = mx + c $$
🌟 What Does y = mx + c Mean?
- $ m $ is the gradient (slope), which determines the steepness of the line.
- $ c $ is the y-intercept, where the line crosses the y-axis.
🌟 How Does y = mx + c Look on a Graph?
The graph of a linear equation always forms a straight line. Linear equations are fundamental in algebra and widely used in real-life applications.
What is the Gradient (m) of Linear Equations? (0:25)
$ m $ is the gradient (also called the slope) of a linear equation. It determines the steepness and direction of the line.
🌟 What Does m in y = mx + c Mean?
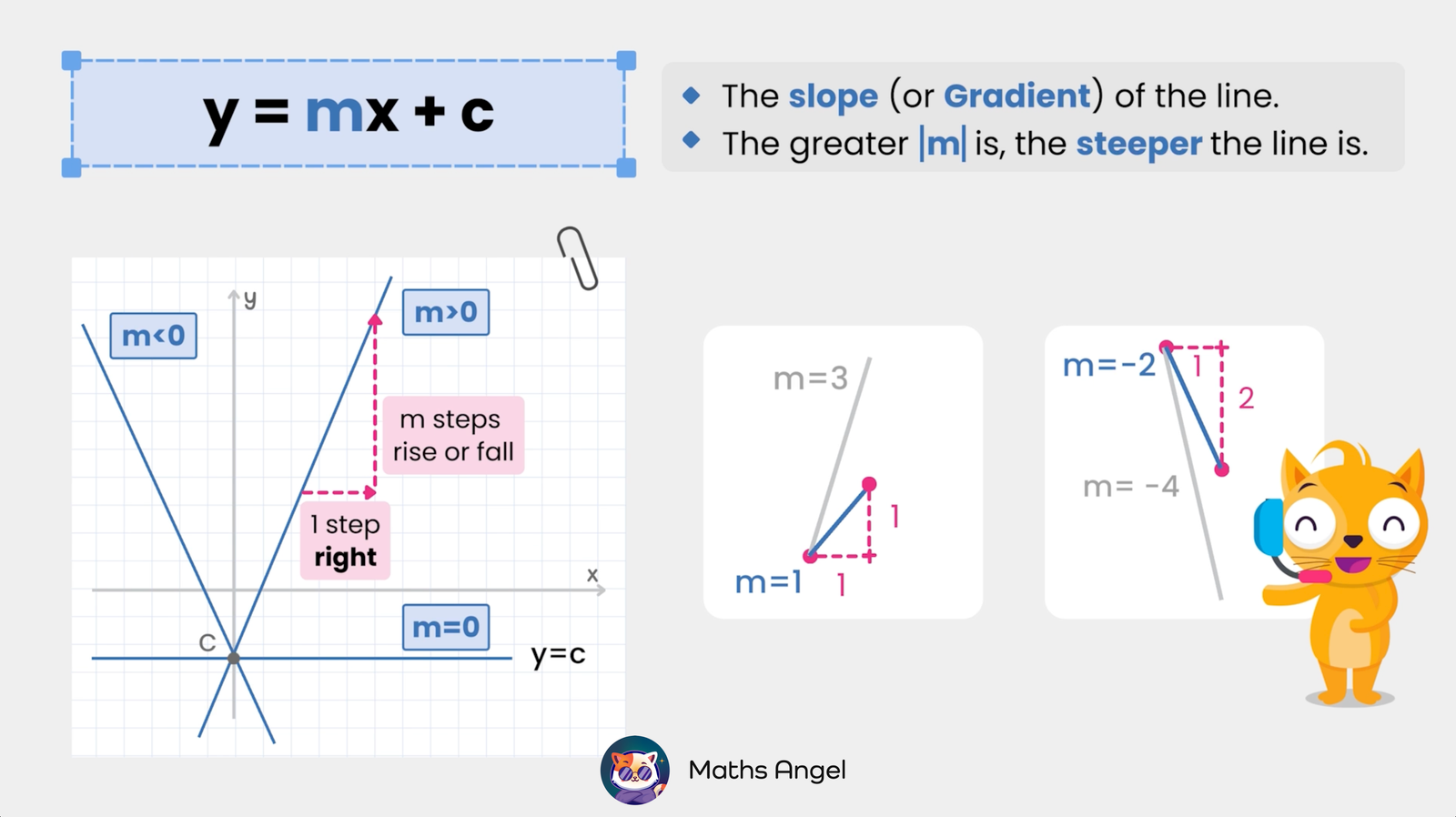
The gradient $ m $ tells us how much the value of $ y $ changes when $ x $ increases by 1.
- If $ m > 0 $, the line slopes upward (from left to right)
- If $ m < 0 $, the line slopes downward (from left to right)
- If $ m = 0 $, the line is horizontal
- The greater the absolute value of $ m $, the steeper the line.
Examples:
- When $ m = 1 $, the line rises 1 unit up for every 1 unit right.
- When $ m = 3 $, the line rises 3 units up for every 1 unit right.
- When $ m = -2 $, the line falls 2 units down for every 1 unit right.
How to Find Y-Intercept of a Linear Equation? (1:30)
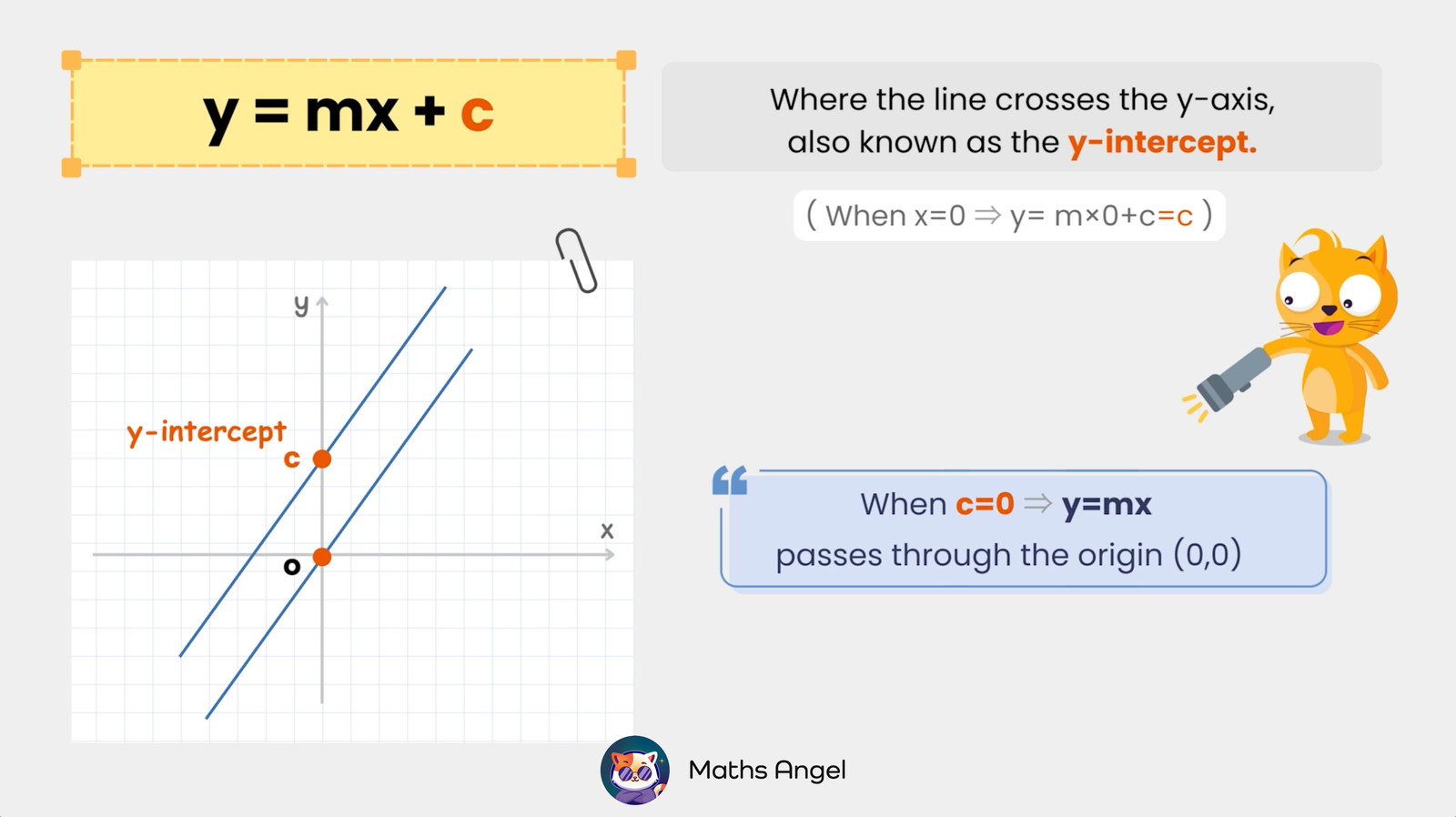
The y-intercept of a linear equation is the point where the line crosses the y-axis.
It is found by setting $ x = 0 $ in the equation:
$$ y = m \cdot 0 + c = c $$
This means the y-intercept at the point $ (0, c) $.
The y-intercept determines where the line starts on the y-axis, shifting the line up or down without changing its slope.
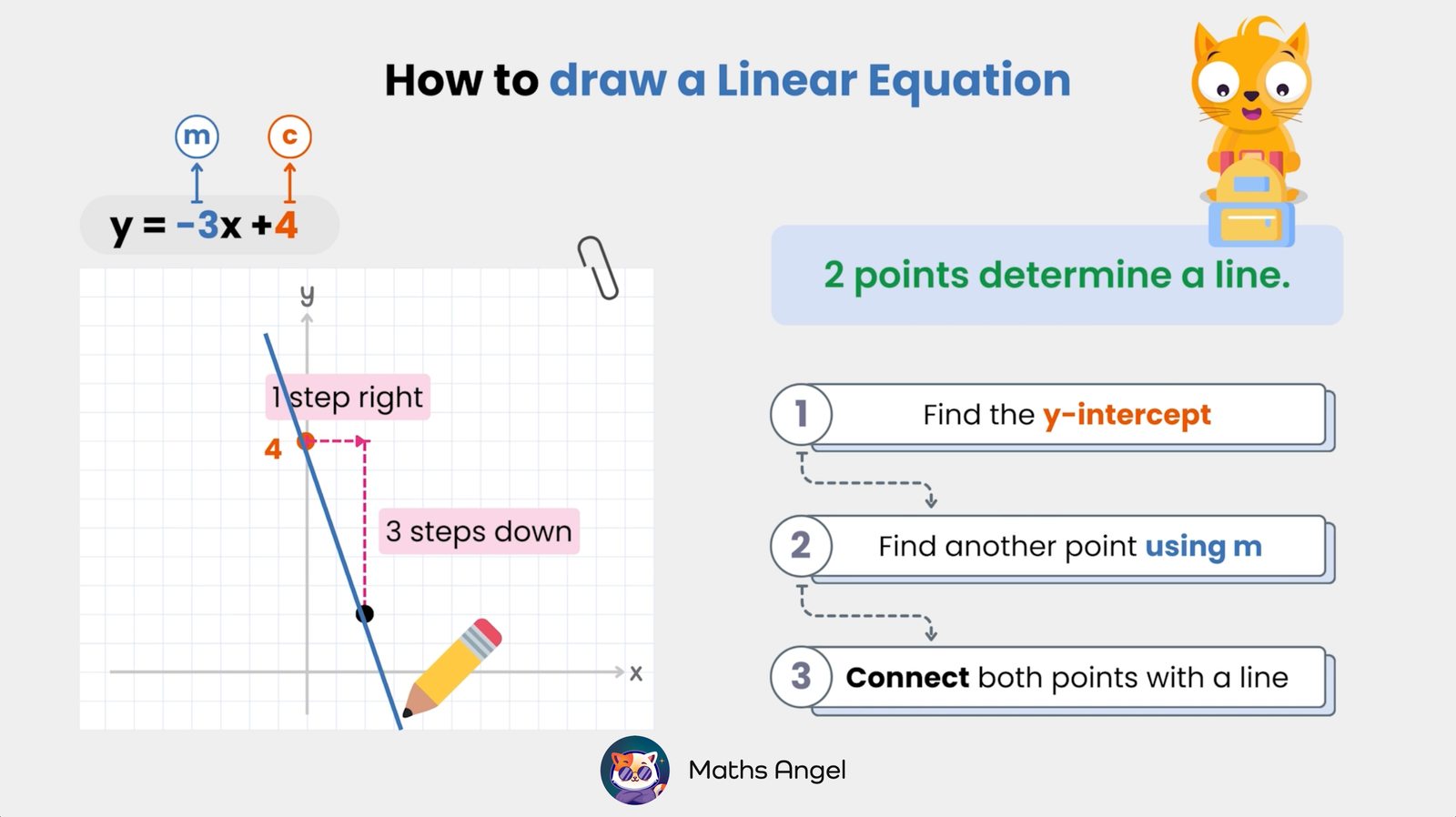
How to Draw a Linear Equation? (2:05)
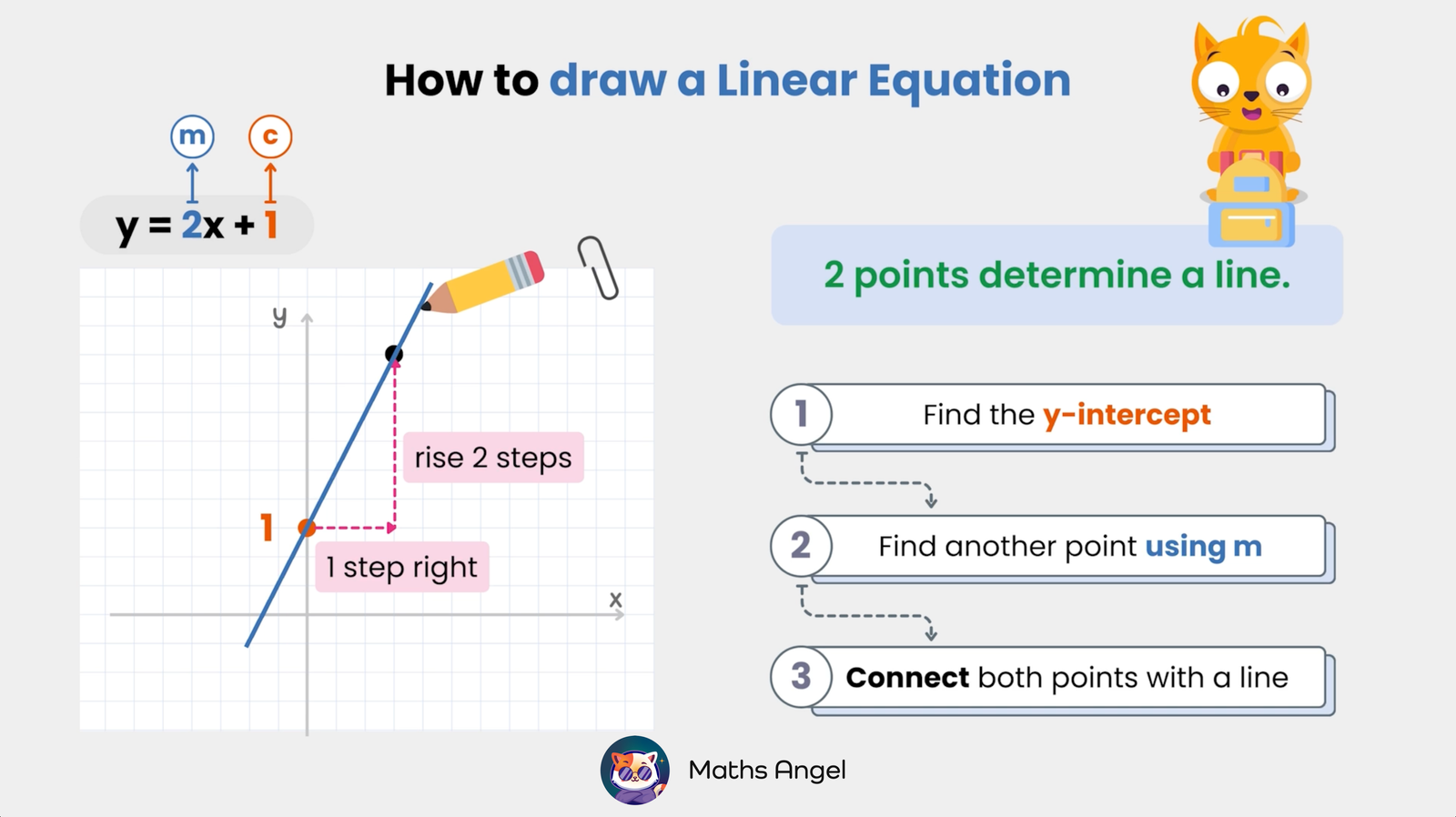
If you know the equation, you can draw the straight line by finding two points on it.
For example, given $ y = 2x + 1 $:
- Step 1: Find the y-intercept.
When $ x = 0 $, $ y = 2 \cdot 0 + 1 = 1 $. So, the first point is $ (0,1) $. - Step 2: Find another point using the gradient.
Since $ m = 2 $, this means that starting from the y-intercept, moving 2 steps up and 1 step to the right, you find a second point: $ (1,3) $. - Step 3: Connect both points with a straight line.
Use a ruler to draw a straight line through the two points. Extend the line in both directions to represent the full equation.
📂 Flashcards: Y-Intercept and Gradient of a Straight Line
🍪 Quiz: Test Your Skills with Y-Intercept and Gradient
🎩 Stuck on Linear Equations? Try AI Math Solver
Need math help? Chat with our AI Math Solver at the bottom right — available 24/7 for instant answers.
