Convert Recurring Decimals to Fractions

🎬 Video Tutorial

(0:01) How to Convert Recurring Decimals to Fractions: Start by setting the recurring decimal as ‘$x$’, then use multiplication to align the repeating parts, making subtraction possible to eliminate them.
(0:10) Turn One-Digit Recurring Decimal to Fraction (Example): Let $x = 0.333\ldots$; multiply by 10 to get $10x = 3.333\ldots$ Subtract the equations to find $9x = 3$. So $x = \large \frac{1}{3}$.
(0:46) Turn Two-Digit Recurring Decimal to Fraction (Example): Set $x = 0.4545\ldots$, multiply by 100 to get $100x = 45.4545\ldots$ Subtract the 2 equations to find $99x = 45$. So $x =\large \frac{5}{11}$.

📂 Revision Cards

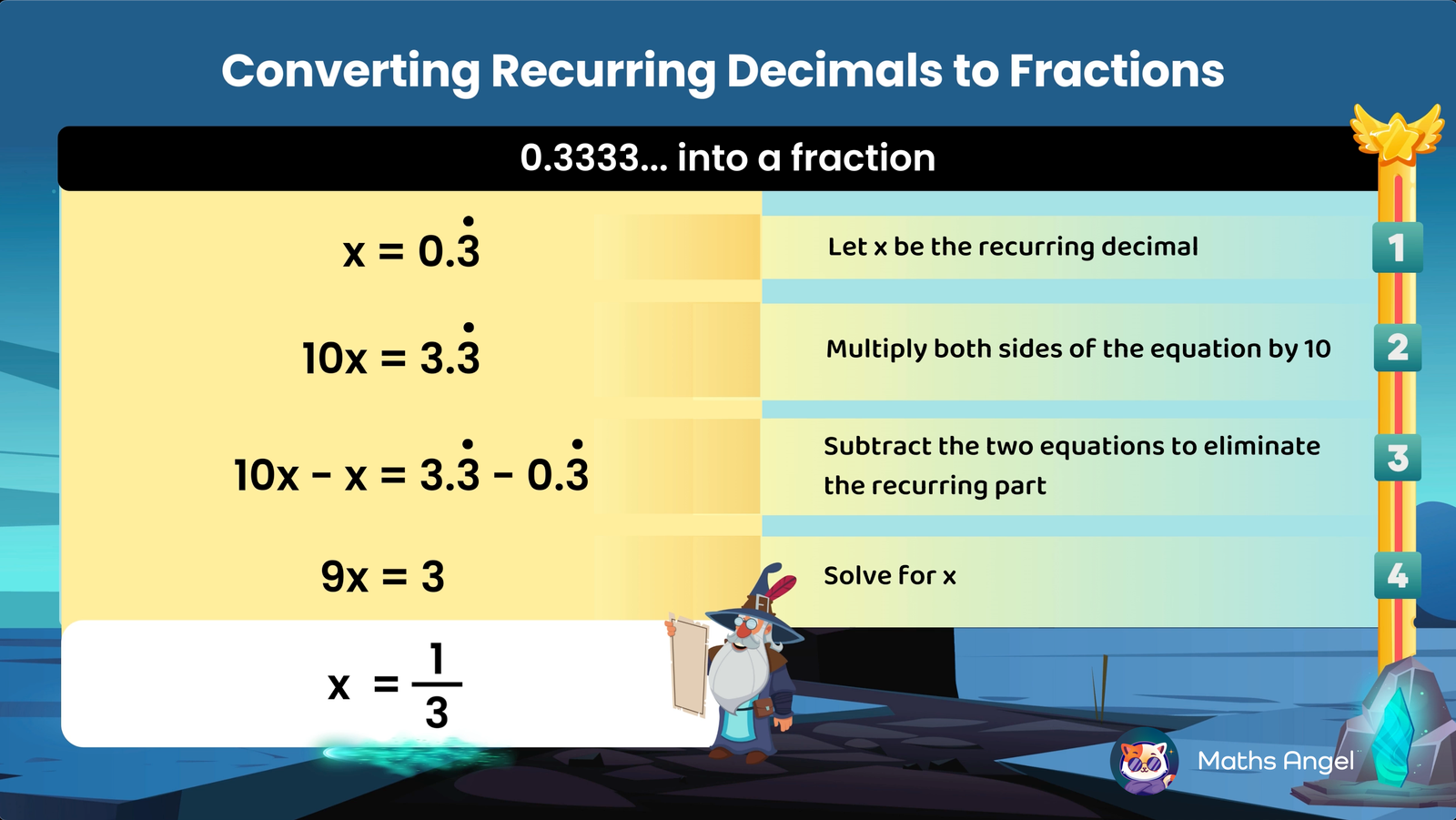
Step-by-step guide converting 0.333 recurring decimal to fraction 1/3 by multiplying by 10, subtracting equations, and solving for x. — 1) Converting Recurring Decimals to Fractions

Converting the recurring decimal 0.4545... into a fraction, multiplying both sides by 100, subtracting the equations, and solving for x = 5/11. — 2) Converting Recurring Decimals to Fractions

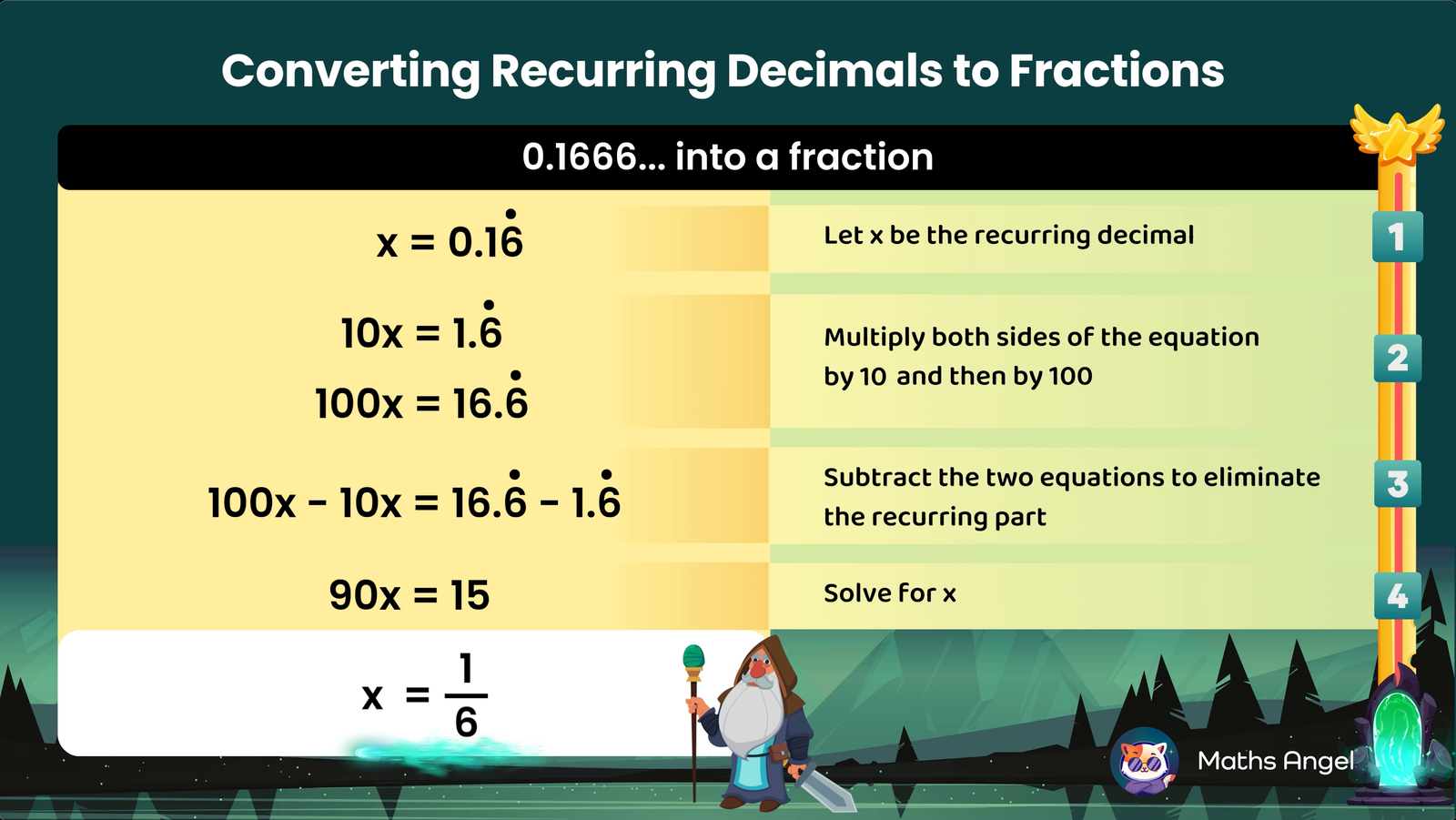
Converting the recurring decimal 0.1666... into a fraction, showing multiplication by powers of 10, subtraction of equations, and solving to get 1/6. — 3) Converting Recurring Decimals to Fractions

🍪 Quiz Time - Practice Now!

Membership Required

You must be a member of Math Angel Plus or Math Angel Unlimited to view this content.

Already a member? Log in here

🎩 AI Math Solver (ChatCat)

Need math help? Chat with our AI Math Solver at the bottom right!

Previous Lesson

0 0 votes

Article Rating

0 Comments

Newest

Oldest Most Voted

Inline Feedbacks

View all comments

Leave a Comment Cancel reply