{results_count} Math videos for {phrase}
Displaying {results_count} results of {results_count_total}
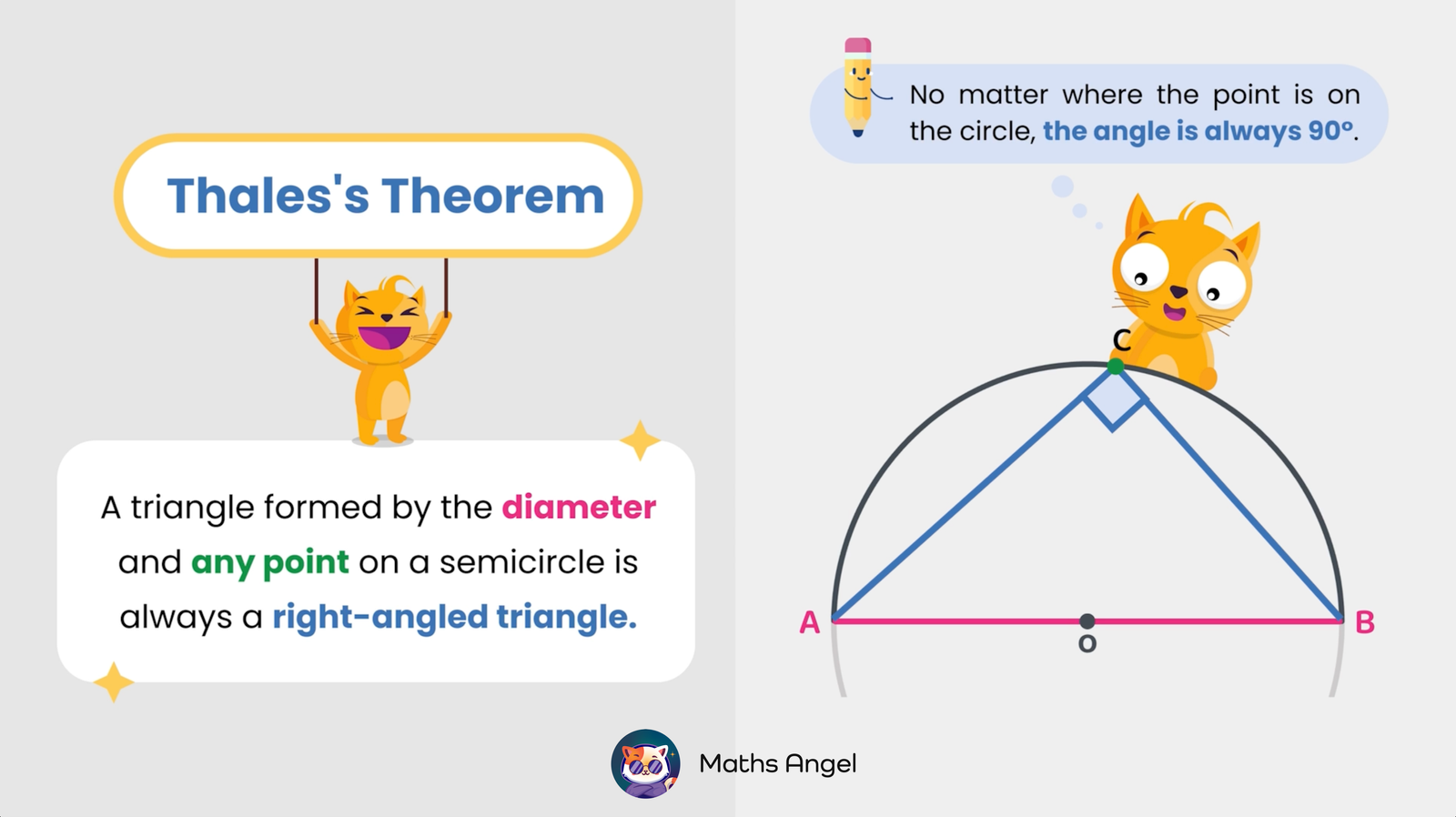
Thales’ Theorem
🎬 Video: Thales' Theorem Definition and Application
What is Thales' Theorem? (0:01)
Thales’s theorem states that if a triangle is formed using the diameter of a circle and any point on the semicircle, it will always be a right-angled triangle.
In the diagram, AB is the diameter of the circle, and C is a point on the semicircle. No matter where C is, the angle at C is always 90°.
How to Apply Thales' Theorem? (0:37)
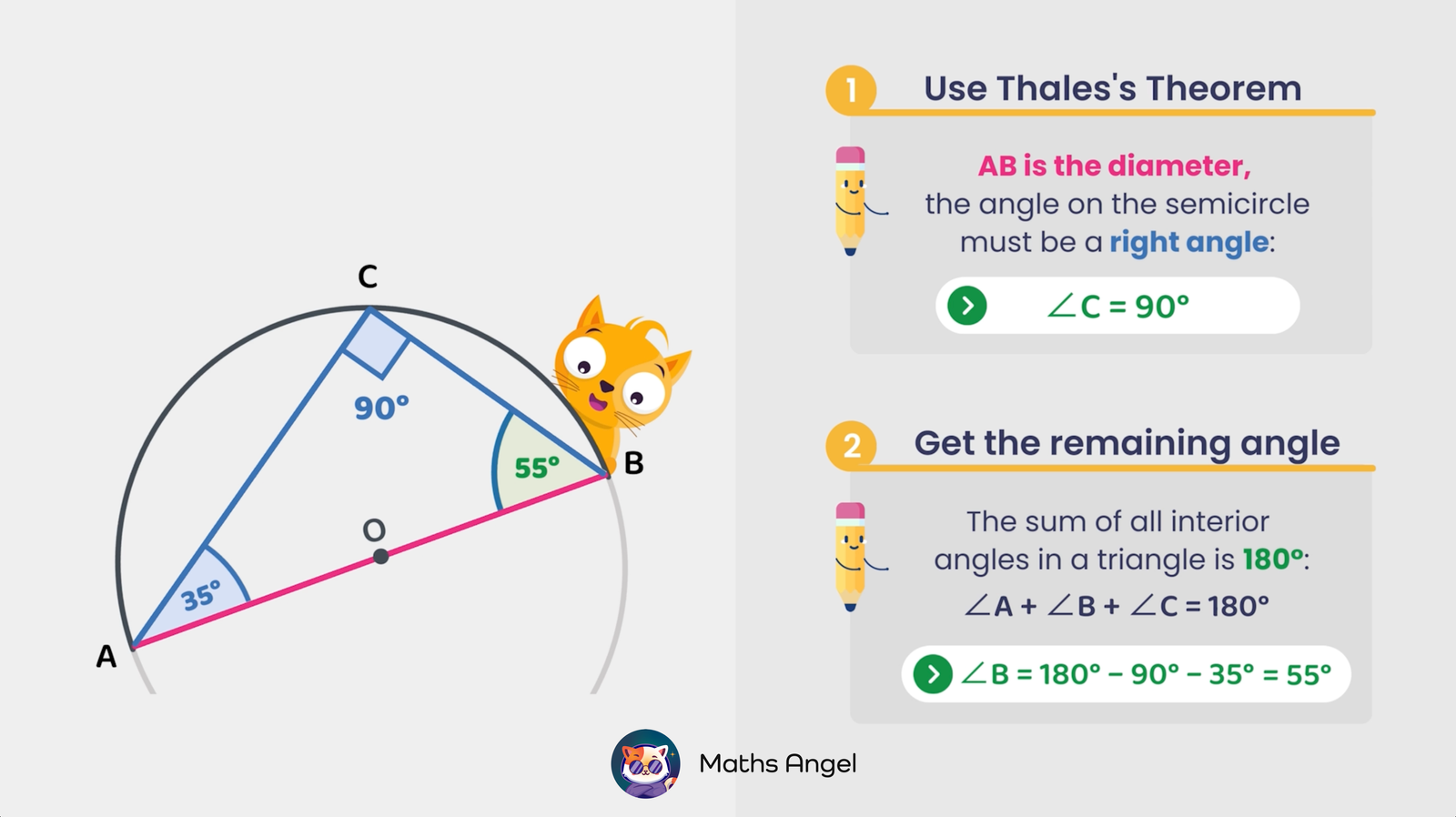
- Step 1: Use Thales’s Theorem
Since $AB$ is the diameter, and vertex $C$ is a point on the semicircle. Thus,
$$ \angle C = 90^\circ $$
- Step 2: Find the Remaining Angle
The sum of all angles in a triangle is:
$$ \angle A + \angle B + \angle C = 180^\circ $$
Since we know $ \angle C = 90^\circ $ and $ \angle A = 35^\circ $, we can find $ \angle B $:
$$ \angle B = 180^\circ – 90^\circ – 35^\circ = 55^\circ $$
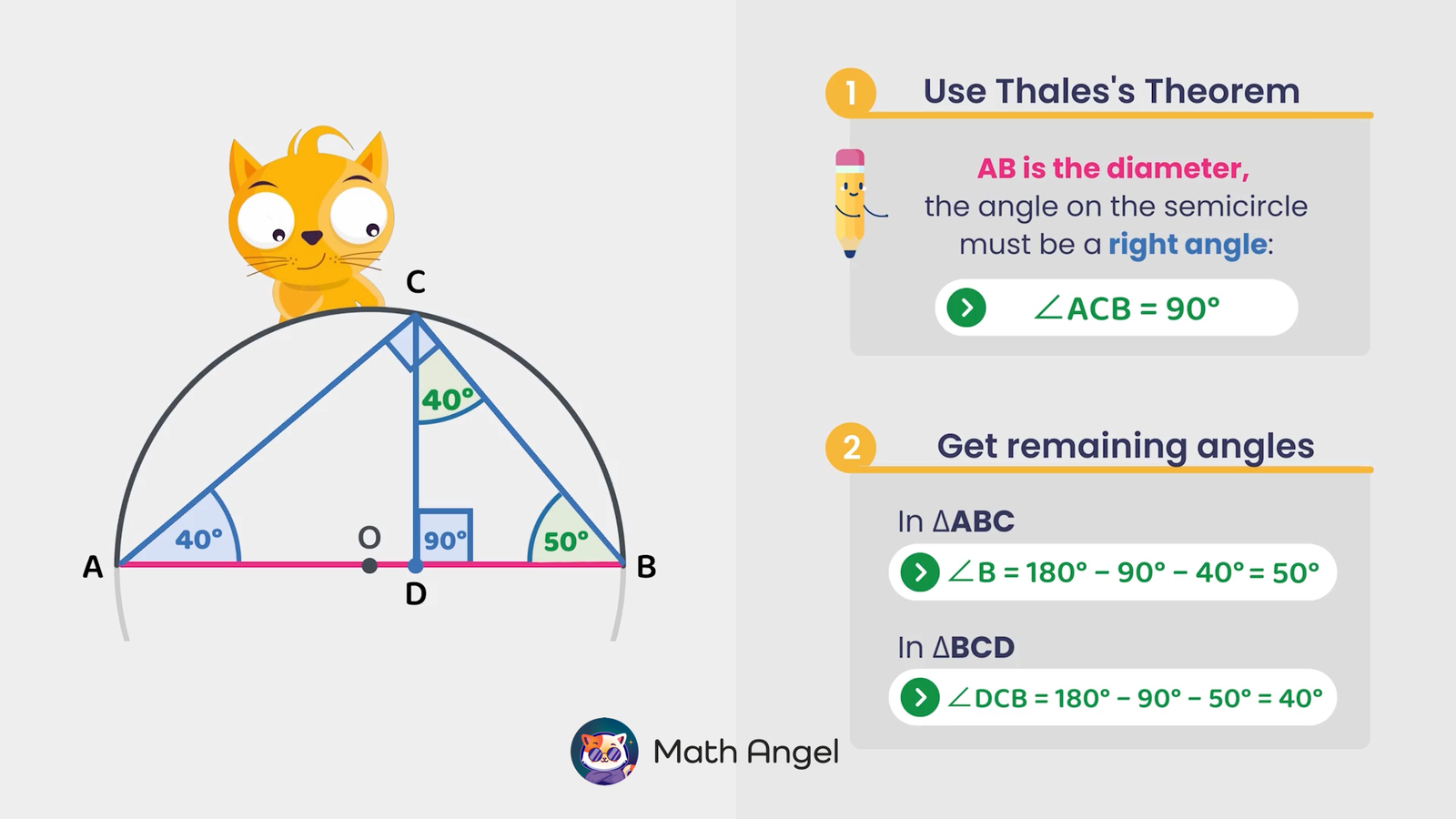
📂 Flashcards: Thales' Theorem Examples with Triangles
🍪 Quiz: Test Your Skills with Thales' Theorem
0%
🎩 Curious About Circle Geometry? Try AI Math Solver
Need math help? Chat with our AI Math Solver at the bottom right — available 24/7 for instant answers.
5
1
vote
Article Rating
0 Comments
Newest
Oldest
Most Voted
Inline Feedbacks
View all comments

