Previous Lesson

Rational Numbers and their location on a Number Line

Numbers and Decimals Rational Numbers and their location on a Number Line

🎬 Video Tutorial

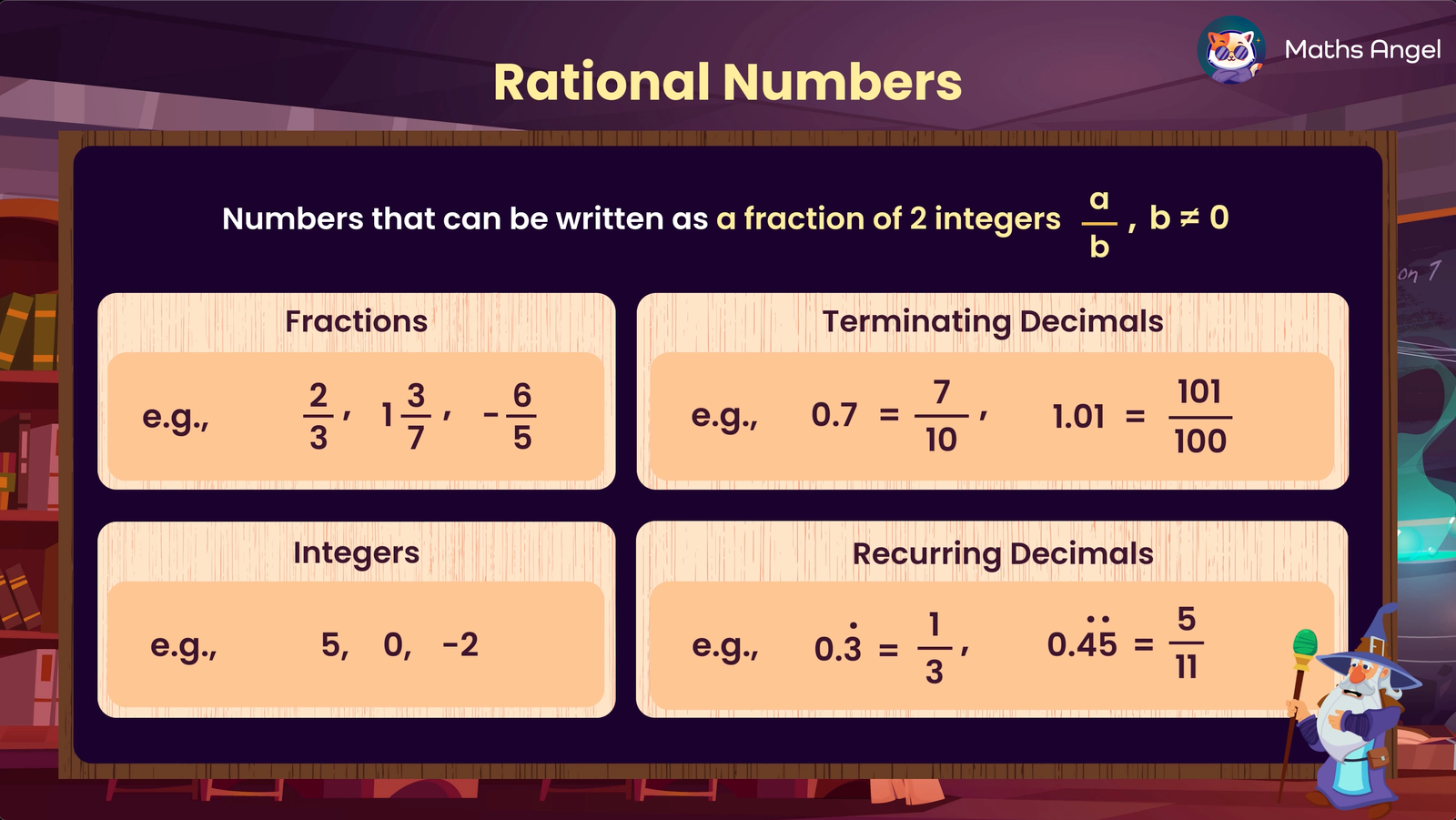
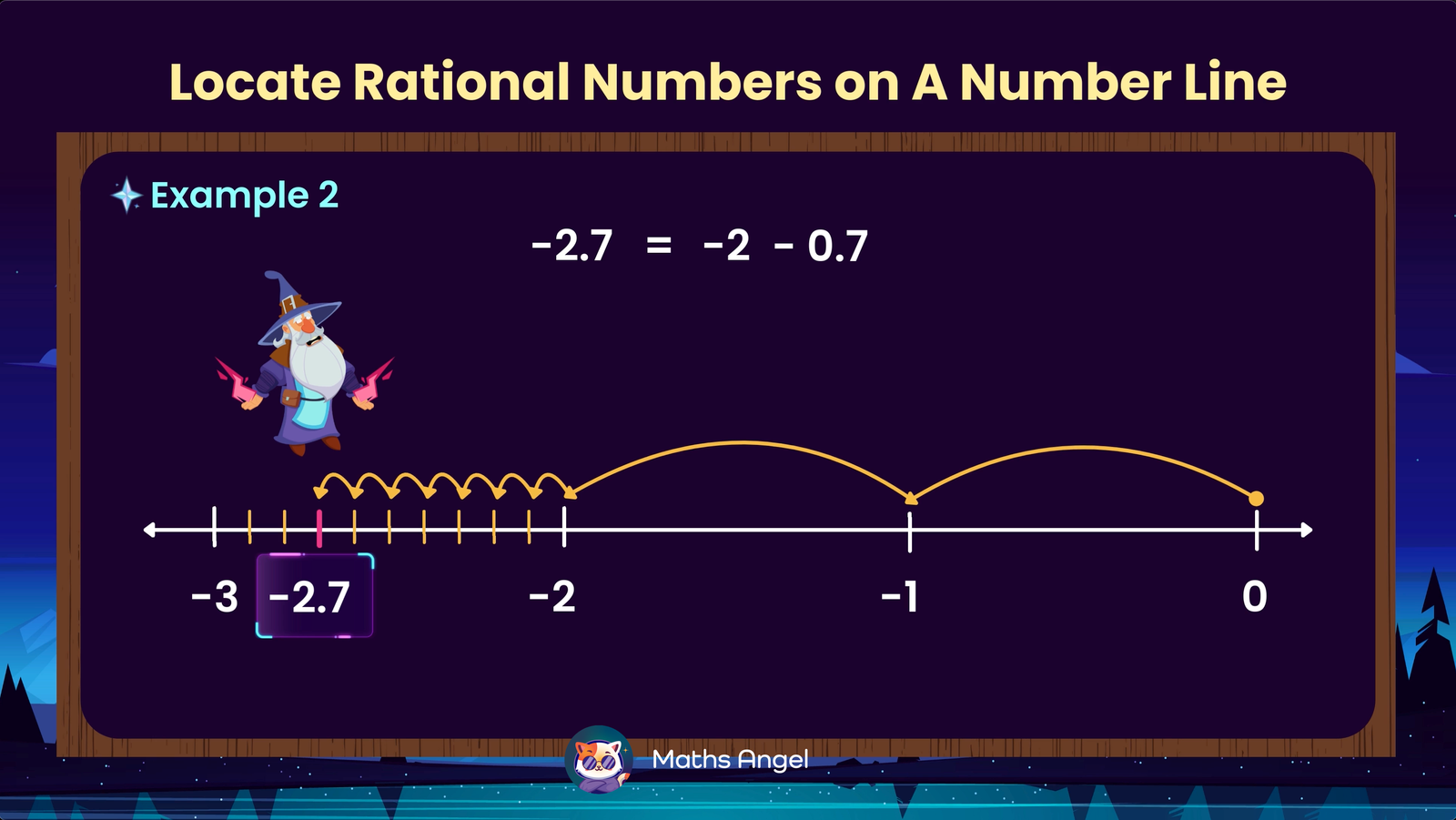
(0:01) What Are Rational Numbers?: Rational numbers are numbers that can be written as a fraction of two integers (a/b) where b ≠ 0.
(0:17) Examples of Rational Numbers: Fractions like 2/3, integers like -2, terminating decimals like 0.7, and recurring decimals like 0.3 recurring (1/3) are all rational numbers.
(1:13) What are Irrational Numbers: Irrational numbers cannot be expressed as a fraction and have non-terminating, non-repeating decimals. For example, $\pi$ and $\sqrt{2}$ .
(2:21) Locating Rational Numbers on a Number Line: Start at 0, move the whole number part first, and then move the fractional part.

📂 Revision Cards

Rational numbers explained with examples showing that fractions, integers, terminating decimals, and recurring decimals are rational numbers. — 1) Rational Numbers

Explaining that irrational numbers are non-terminating decimals without repeating patterns, with examples like ? and ?2. — 2) Irrational Numbers

Locating the rational number and fraction 11/3 on a number line by dividing it into 3 and 2/3, demonstrating on number line. — 3) Rational Numbers on a Number Line

Locating the rational number and decimal -2.7 on a number line, shown as -2 minus 0.7. — 4) Rational Numbers on a Number Line

🍪 Quiz Time - Practice Now!

Membership Required

You must be a member of Math Angel Plus or Math Angel Unlimited to view this content.

Already a member? Log in here

🎩 AI Math Solver (ChatCat)

Need math help? Chat with our AI Math Solver at the bottom right!

Previous Lesson

0 0 votes

Article Rating

0 Comments

Newest

Oldest Most Voted

Inline Feedbacks

View all comments

Post a comment

Leave a Comment Cancel reply