Multiplying and Dividing Square Roots
Table Of Contents
🎬 Math Angel Video: How to Multiply and Divide Square Roots
Multiplying and Dividing Square Roots (Formulas)
⏩️
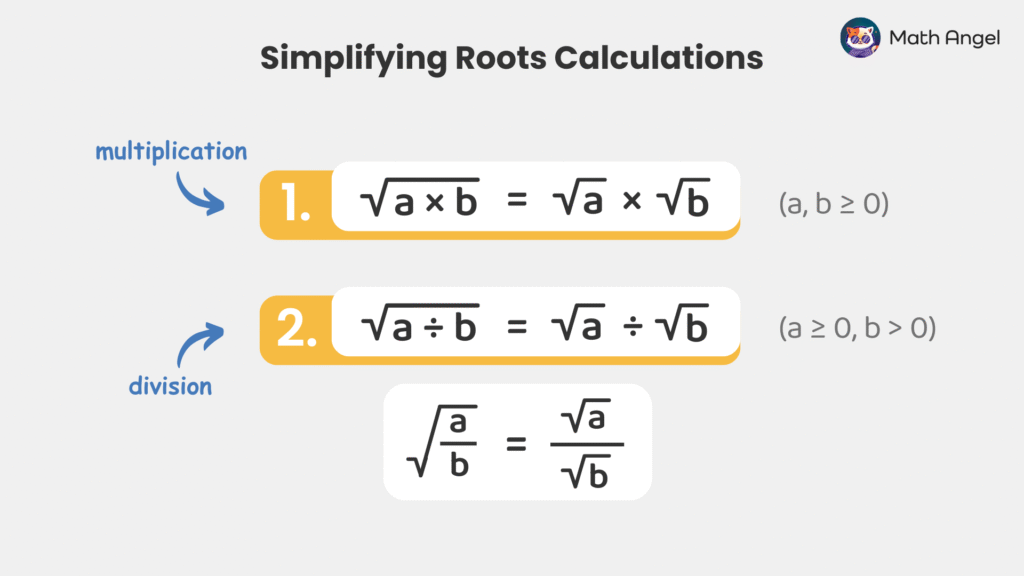
🛎️ Multiplication Rule for Square Roots
If $ a \geq 0 $ and $ b \geq 0 $, then:
$$ \sqrt{a} \times \sqrt{b} = \sqrt{a \times b} $$
🛎️ Division Rule for Square Roots
If $ a \geq 0 $ and $ b > 0 $, then:
$$ \sqrt{a} \div \sqrt{b} = \sqrt{a \div b} $$
or equivalently,
$$ \frac{\sqrt{a}}{\sqrt{b}} = \sqrt{\frac{a}{b}} $$
By following these rules, you can efficiently work with square roots in calculations.
How to Multiply Square Roots? (Examples)
⏩️
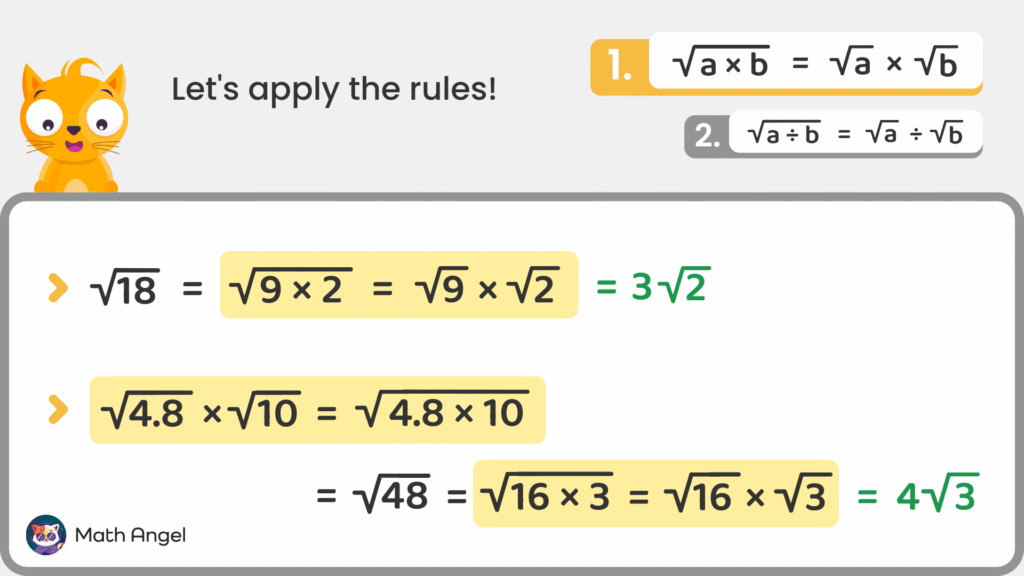
The multiplication rule states that for any non-negative numbers $ a $ and $ b $:
$$ \sqrt{a} \times \sqrt{b} = \sqrt{a \times b} $$
You can use the formula from left to right or from right to left. This helps you simplify square roots.
🛎️ Example 1: Simplifying Surds
$$ \sqrt{18} = \sqrt{9 \times 2} = \sqrt{9} \times \sqrt{2} = 3\sqrt{2} $$
🛎️ Example 2: Multiplying Surds
- First, we apply the multiplication rule:
$$ \sqrt{4.8} \times \sqrt{10} = \sqrt{4.8 \times 10} = \sqrt{48} $$
- Now, we reverse the rule to simplify:
$$ \sqrt{48} = \sqrt{16 \times 3} = \sqrt{16} \times \sqrt{3} = 4\sqrt{3} $$
- This example is useful because it demonstrates the square root multiplication rule in both directions.
How to Divide Square Roots? (Examples)

⏩️
The division rule states that for any non-negative numbers $ a $ and positive number $ b $:
$$ \sqrt{a} \div \sqrt{b} = \sqrt{a \div b} $$
or equivalently,
$$ \frac{\sqrt{a}}{\sqrt{b}} = \sqrt{\frac{a}{b}} $$
By applying this rule, we can simplify square roots effectively.
🛎️ Example 1: Simplifying Surds
$$ \sqrt{36 \div 25} = \sqrt{36} \div \sqrt{25} = 6 \div 5 = 1.2 $$
🛎️ Example 2: Dividing Square Roots
$$ \frac{\sqrt{50}}{\sqrt{2}} = \sqrt{\frac{50}{2}} = \sqrt{25} = 5 $$
🍪 Quiz: Practice Simplifying Surds and Square Roots
🎩 Stuck on Surds or Square Roots? Try AI Math Solver
Need math help? Chat with our AI Math Solver at the bottom right — available 24/7 for instant answers.

