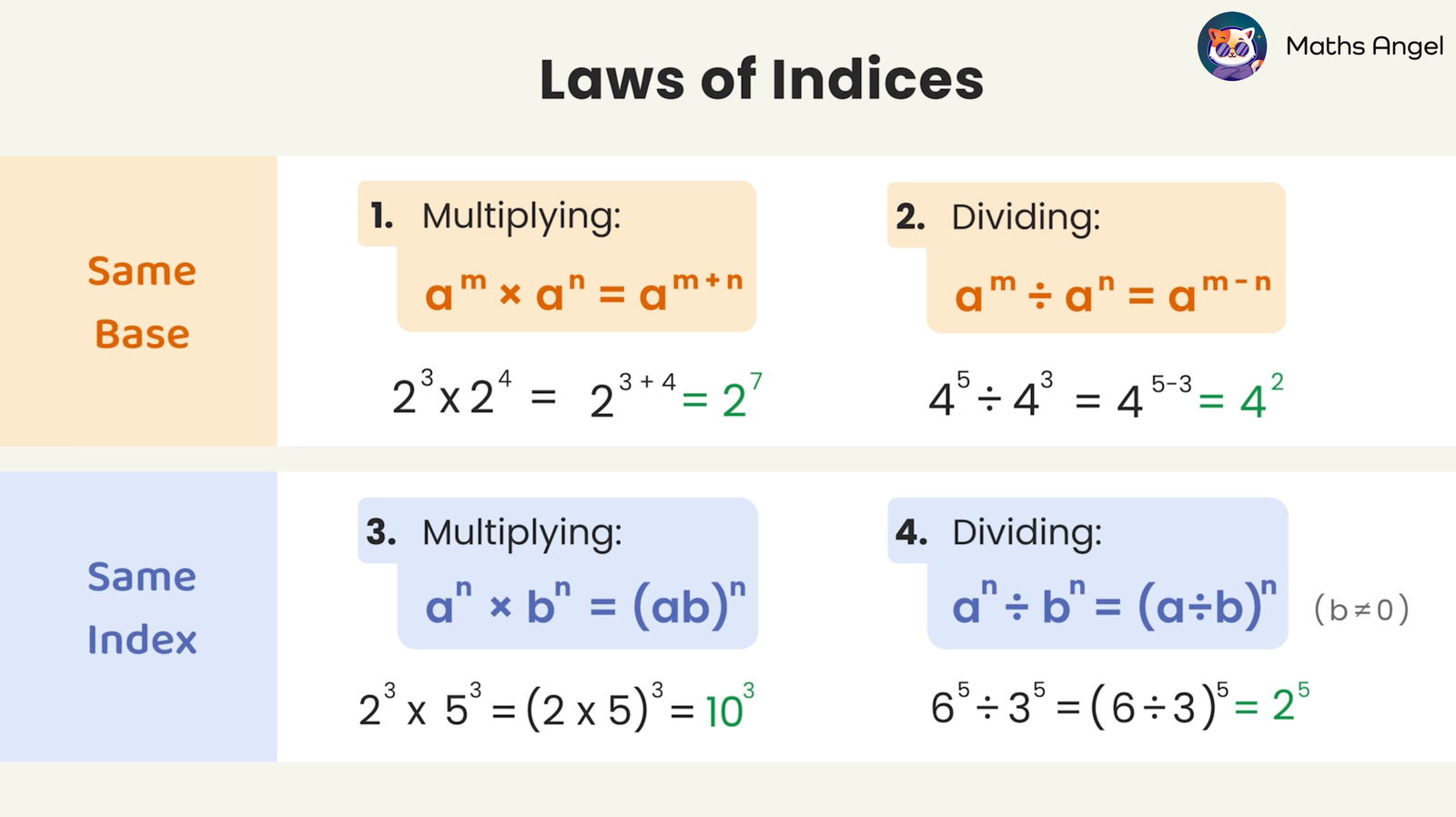
Laws of Indices (Same Base, Same Indices)

🎬 Video Tutorial

Membership Required

You must be a member of Math Angel Plus or Math Angel Unlimited to view this content.

Join Now

Already a member? Log in here

(0:01) What are Laws of Indices?: Laws of Indices are rules that simplify calculations involving powers of numbers.
(0:21) Multiplying Indices with Same Base: For multiplication, add the indices ($a^m \times a^n = a^{m+n}$). For example, $2^3 \times 2^4 = 2^{3+4} = 2^7$.
(1:10) Dividing Indices with Same Base: For division, subtract the indices ( $a^m \div a^n = a^{m-n}$). For example, $4^5 \div 4^3 = 4^{5-3} = 4^2$.
(2:02) Multiplying Indices with Same Index: For multiplication, multiply the bases and keep the index ($a^m \times b^m = (a \times b)^m$). For example, $2^3 \times 5^3 = (2 \times 5)^3 = 10^3$.
(2:56) Dividing Indices with Same Index: For division, divide the bases and keep the index ($\frac{a^m}{b^m} = \left(\frac{a}{b}\right)^m$). For example, $\frac{6^5}{3^5} = \left(\frac{6}{3}\right)^5 = 2^5$.

📂 Revision Cards

Laws of indices chart showing rules for multiplying and dividing powers with the same base and same index, with examples for both operations. — Law of Indices Summary

🍪 Quiz Time - Practice Now!

Laws of Indices (Same Base or Same Indices)

1 / 6

Q: What is $2^3 \times 2^4$?

$2^{12}$

$2^7$

$2^6$

$2^8$

2 / 6

Q: What is $4^5 \div 4^3$?

$4^3$

$4^{15}$

$4^2$

$4^8$

3 / 6

Q: What is $10^5 \div 10^2$?

$10^2$

$10^3$

$10^2.5$

$10^1$

4 / 6

Q: Simplify $0.2^5 \times 5^5$.

$5$

$1$

$(0.2 \times 5)^5$

$0.1^5$

5 / 6

Q: Simplify $5^4 \div 5^6$.

$5^{-3}$

$5^0$

$5^{-2}$

$5^2$

6 / 6

Q: What is $2^4 \times 2^2 \times 2^3$?

$2^{10}$

$2^8$

$2^9$

$2^7$

Your score is

The average score is 100%

🎩 AI Math Solver (ChatCat)

Need math help? Chat with our AI Math Solver at the bottom right!

Log In

Join Now | Lost Password?

Numbers and Decimals