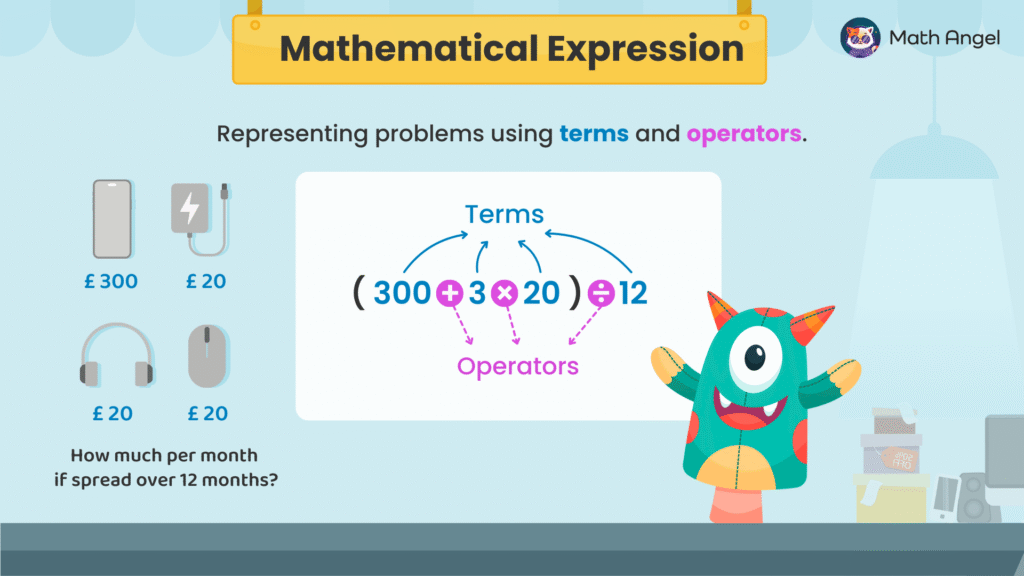
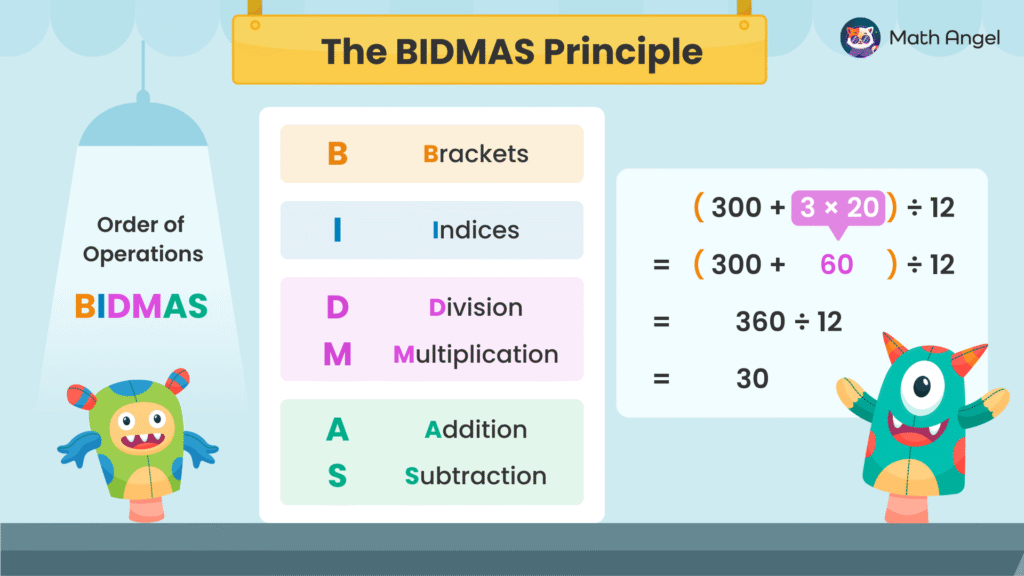
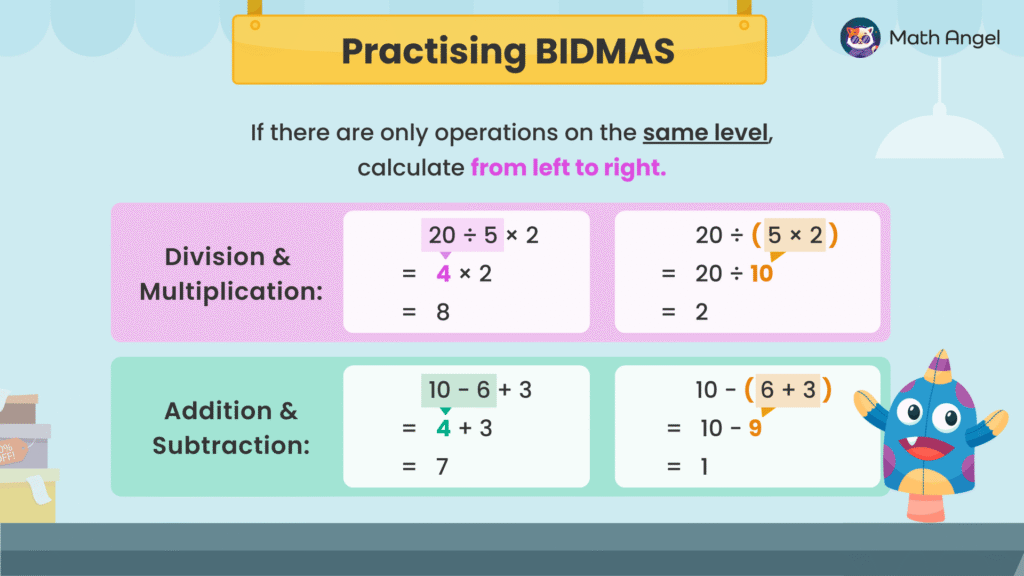
A mathematical expression is a combination of numbers, variables, and operators. It does not have an equals sign (=), unlike an equation.
For example, $$(300 + 3 \times 20) \div 12$$
Parts of a Mathematical Expression:
Mathematical expressions help us write real-life problems in a structured way so we can calculate them easily!
The order of operations is the rule that tells us the correct sequence to follow when solving a mathematical expression. We can use BIDMAS to remember the order:
For example:
$$ (300 + 3 \times 20) \div 12 $$
Brackets come first, but inside the brackets, you must still follow BIDMAS rules. Since multiplication (M) comes before addition (A), you do multiplication first:
$$ 3 \times 20 = 60 $$
So the expression simplifies to:
$$ (300 + 60) \div 12 $$
Solve the addition inside the brackets:
$$ 300 + 60 = 360 $$
Finally, you divide:
$$ 360 \div 12 = 30 $$
If a calculation only contains operations of the same level (such as only division and multiplication, or only addition and subtraction), solve from left to right.
However, if there are brackets, you must solve inside the brackets first.
💡 Example 1:
$$ 20 \div 5 \times 2 = 4 \times 2 = 8 $$
$$ 20 \div (5 \times 2) = 20 \div 10 = 2 $$
💡 Example 2:
$$ 10 – 6 + 3 = 4 + 3 = 7 $$
$$ 10 – (6 + 3) = 10 – 9 = 1 $$
If there are multiple sets of brackets, solve the innermost brackets first, then work outwards.
For example,
$$
\begin{aligned}
50 – (2 \times (2 + 4 \times 5) – 3) &= 50 – (2 \times (2 + 20) – 3) \\[1em]
&= 50 – (2 \times 22 – 3) \\[1em]
&= 50 – (44 – 3) \\[1em]
&= 50 – 41 \\[1em]
&= 9
\end{aligned}
$$
Need math help? Chat with our AI Math Solver at the bottom right — available 24/7 for instant answers.
